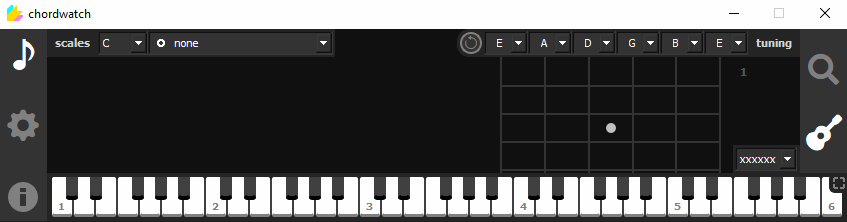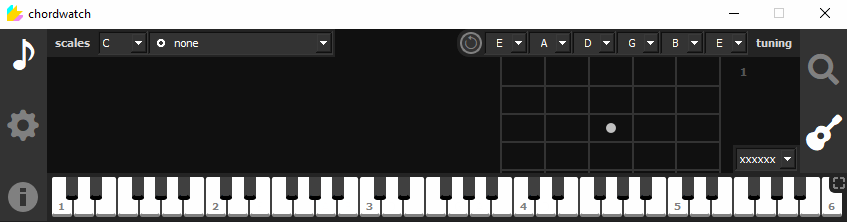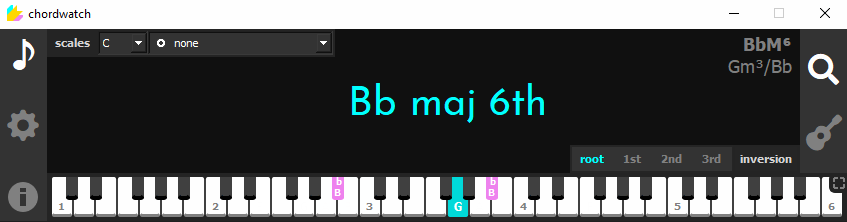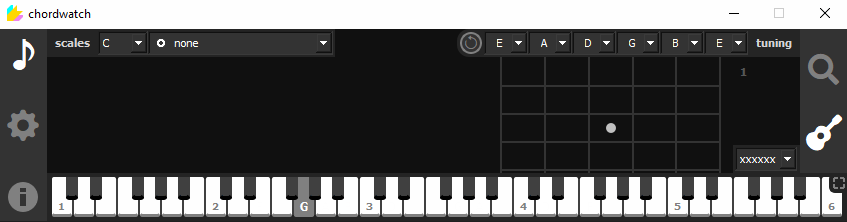
Music Interval Ear Training: Interactive Piano Training Tool
🎵 This interactive musical tool makes it easy to play and hear different
music intervals on the piano, both simple intervals and
compound intervals.
You'll be able to play the 5 interval qualities:
minor, major, augmented, diminished and perfect .
Click on any of the interval buttons below to play that interval on the piano.
Each click of an interval button will select a different root note at random.
This makes for an easy ear training tool to train your ears with different
intervals and how they sound.
🎲 Plus, you'll find an interval identification game to test and train your
music interval recognition skills.
You may also like the intervals chart and the
interval calculator.
note display:
play mode:
-
C♯
D♭ - D
-
D♯
E♭ - E
- F
-
F♯
G♭ - G
-
G♯
A♭ - A
-
A♯
B♭ - B
-
C♯
D♭ - D
-
D♯
E♭ - E
- F
-
F♯
G♭ - G
-
G♯
A♭ - A
-
A♯
B♭ - B
Simple Intervals
Compound Intervals
🎲 Interval Identification Game
Use this little game to test your music interval recognition skills. The game will play a random simple interval and your task is to guess which interval that is.
Your guess ↷
✨⌨️ You can control this music interval identification game with a computer keyboard. ⬅️ Left arrow to play an interval again, ➡️ right arrow to skip to the next one, and numbers keys for your guess (1️⃣, 2️⃣, 3️⃣, 4️⃣, 5️⃣ or 6️⃣).
Learning about music intervals
In music, intervals are simply the distance between two notes. Each interval has a distinct sonic quality and intervals form an important basis for music and how harmonies and melodies sound.
Intervals are named with a quality and a number. There are 5 possible interval qualities :
- Minor: minor 2nd, minor 3rd, minor 7th,...
- Major: major 2nd, major 3rd, major 7th,...
- Perfect: perfect 4th, perfect 5th, perfect octave (often just called octave), perfect unison (often just called unison).
- Diminished: diminished 3rd, diminished 5th,...
- Augmented: augmented 3rd, augmented 5th,...
Simple vs Compound Intervals
Intervals can be simple or compound. Simple intervals fit within one octave (8 degrees), while compound intervals span multiple octaves (more than 8 degrees), usually only a maximum of two octaves.
Interval Names
To determine an interval name, we can first determine its number by counting the number of natural notes between the two notes of the interval, including the starting and ending notes. This will give us the number of degrees between the two notes. For example, from C to E is an interval of a 3rd because they span 3 natural notes (3 degrees): C - D - E.
For the interval quality, 4ths and 5ths are usually perfect, and 2nds, 3rds, 6ths and 7ths are usually either minor or major.
To definitively determine an interval name with its quality, we can count the number of half steps (semitones) between the two notes, without counting the starting note.
Below you'll find a table with interval names and their number of half steps (semitones):
| Name | Half steps |
|---|---|
| Minor 2nd (m2) | 1 |
| Major 2nd (M2) | 2 |
| Minor 3rd (m3) | 3 |
| Major 3rd (M3) | 4 |
| Perfect 4th (P4) | 5 |
| Tritone (Augmented 4th (A4) or Diminished 5th (d5)) | 6 |
| Perfect 5th (P5) | 7 |
| Minor 6th (m6) | 8 |
| Major 6th (M6) | 9 |
| Minor 7th (m7) | 10 |
| Major 7th (M7) | 11 |
| Octave (P8) | 12 |
| Minor 9th (m9) | 13 |
| Major 9th (M9) | 14 |
| Minor 10th (m10) | 15 |
| Major 10th (M10) | 16 |
| Perfect 11th (P11) | 17 |
| Augmented 11th (A11) or Diminished 12th (d12) | 18 |
| Perfect 12th (P12) | 19 |
| Minor 13th (m13) | 20 |
| Major 13th (M13) | 21 |
| Minor 14th (m14) | 22 |
| Major 14th (M14) | 23 |
| Fifteenth (aka double octave) (P15) | 24 |
Inverted Intervals (aka reciprocal intervals)
Each interval has an associated inverted or reciprocal interval, when the two notes of the interval are flipped. For example, C to E has an interval of a major third, and E to C, its inverted interval, is of a minor 6th.
An interval number and its inverted interval will always equal 9, so 3 goes with 6 (3 + 6 = 9), 4 with 5 (4 + 5 = 9), 2 with 7 (2 + 7 = 9),...
The inverted interval of a major interval is minor, the inverted interval of a minor interval is major, the inverted interval of a perfect is still perfect and the inverted interval of a diminished is augmented.